Definition of the Derivative

The Slope of a Secant Line

Example:  

Consider the function 

        y = f(x) = x²  

Then the secant line from x = 2 to x = 4 is defined by the the line that joins the two points (2,f(2)) and (4,f(4)).  This line has slope

                            f(4) - f(2)         16 - 4         
        rise/run =                        =                  =   6     
                               4 - 2              4 - 2          

In general, we give the following definition

        

Definition of the Slope of the Secant line Let y = f(x) be a function.  Then the slope of the secant line between x  =  a  and  x  =   b  is                                f(b) - f(a)                                                                                      b - a

The Derivative

If instead of choosing (4,f(4)), we choose (2 + h,f(2 + h)) as our second point we have that the slope of the secant line is

             f(2 + h) - f(2)         f(2 + h) - f(2)               
                                     =                               
               (2 + h) - 2                      h          

                (2 + h)² - 2²           4 + 4h + h² - 4               
          =                             =                                 
                          h                               h          

                 4h + h²                         
          =                       =   4 + h
                      h                           

What happens when we let h approach 0?  Geometrically this is called the slope of the tangent line to f(x) at x = 2.  Analytically, this is called the Derivative of f(x) at x = 2.  For the above example, the limit is 4.  We say that f '(2)  =  4.

 

Definition of the Derivative The Derivative (f '(x) or df /dx) of f(x) at x = c is defined by

Exercises:  Find f ' of the following functions

A.  f(x) = 2x - 1  at x = 2

B.  f(x) = x² at x = 1

C.  f(x) = 1/x  at x = 3

D.  f(x) =  at x = 4

We call the function that takes an x - value to the derivative at the x value the derivative of the function.

Example

Find the derivative of 

                     3
        f(x)  =        
                     x

Solution

We find the limit

Now multiply by the numerator and denominator by x(x + h) to get

Exercises:  Find f '(x) for the following functions

A.  f(x) = x³

B.  f(x) = 4 - x

C.  f(x)  =  

Alternate Form of the Derivative

There is another way to write the derivative.  Since the derivative is the limiting slope of the secant line, we can think as the first point at c be fixed and then second point be a varying value x that tend towards c.  Then the slope of the tangent line will be

If a function is not continuous then the numerator will either not exist or will not approach zero, while the denominator will approach zero.  This leads us to the following theorem.

 

Theorem If f(x) is a differentiable function at x = c then f(x) is continuous at x = c.

The converse of this theorem is not always true.  That is there are continuous functions that are not differentiable.  For example, let 

        f(x)  =  | x |

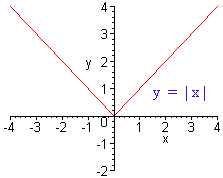
From the graph, we can see that the slope of the secant line will be -1 to the left of 0 and 1 to the right of 0.  At the origin the derivative is undefined even though f(x) is continuous at the origin.

When is a function differentiable?  

Answer:  When it is continuous, has no sharp edges, and the tangent line is not vertical.

Back to the calculus home page

Back to the math department home page

e-mail Questions and Suggestions