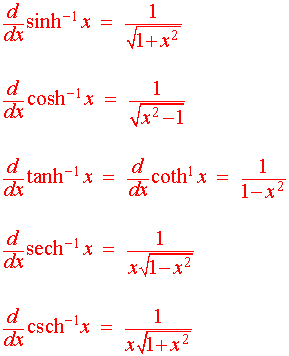Hyperbolic Functions
Definition of the Hyperbolic Functions
We define the hyperbolic functions as follows: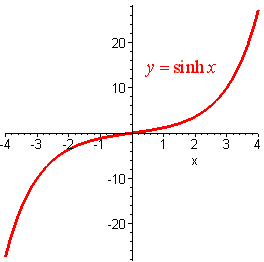
|
ex + e-x
sinh x |
Properties
-
(cosh x)2 - (sinh x)2 = 1
-
d/dx(sinh x) = cosh x
-
d/dx(cosh x) = sinh x
Proof of A
We find
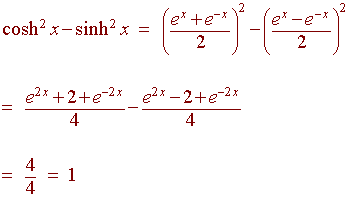
The Derivative of the Inverse Hyperbolic Trig Functions
|
Theorem
|
Proof of the third identity
We have
tanh(arctanh x) = x
Taking derivatives implicitly, we have
d
sech2(arctanh x)
arctanh x = 1
dx
Dividing gives
d
1
arctanh x =
dx
sech2(arctanh x)
Since
cosh2(x) - sinh2(x) =
1
dividing by
cosh2(x), we get
1 - tanh2(x) = sech2(x)
so that
d 1
1
arctanh x =
=
dx 1 - tanh2(arctanh
x) 1 - x2
For the derivative of the inverse sech(x) click here
Integration and Hyperbolic Functions
Now we are ready to use the arc hyperbolic functions for integration
Example:
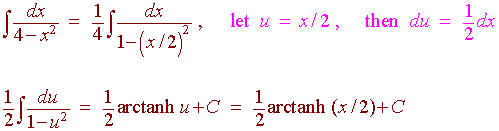
Example
Evaluate
![]()
Solution
Although this is not directly a derivative of a hyperbolic trig function, we
can use the substitution
u = x2
, du = 2x dx
To change the integral to
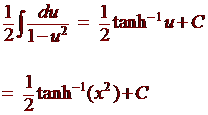
Back to the Math Department Home
e-mail Questions and Suggestions