The Parabola
Algebraic Definition of The Parabola
Recall that the standard equation of the parabola is given by
|
y = a(x - h)2 + k |
If we are given the equation of a parabola
y = ax2 +bx + c
we can complete the square to get the parabola in standard form.
Geometry of the Parabola
We can define a parabola as follows:
|
Geometric Definition of the Parabola Let F be a point on the plane and let y = -p be horizontal line called the directrix. Then the set of points P such that FP is equal to the distance from the line to P is a parabola. |
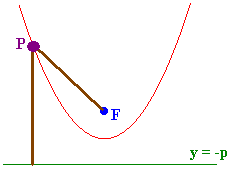
Example
Let
F = (0,2)
and
y = -2
be the directrix.
Then
FP = (x2 + (y - 2)2)1/2
and the distance from P to
the directrix is given by
2 + y
Hence
2 + y = (x2 + (y - 2)2)1/2
squaring both sides,
we get
4 + 4y + y2 = (x2 + (y - 2)2) =
x2 + y2 - 4y + 4
We have
8y = x2
or
y = x2/8
In general if
y = -p
is the equation of the directrix and
V = (h,k)
is the vertex, then the Focus is at the point
F = (h,k + p)
and the equation of the
parabola is
|
y = 1/4p (x - h)2 + k |
Note that vertex will always be half way between the focus and the directrix.
Example:
Find the equation of the parabola with Focus at (1,2) and directrix
y = -4.
Solution
We see that the vertex is at the point
(1, (-4+2)/2) =
(1,-1)
Since the directrix is y = -4, we have
p = 4
so that
1/4p = 1/16
Hence the equation is
y = -1/16 (x - 1)2 - 1
Optics
Why the word focus?
Application 1:
A flashlight.
If a flashlight is to be 3 in in diameter and 2
inches deep, where should
the bulb be placed?
Solution:
If the bulb is placed at the focus then the reflected light rays from the
bulb will all travel in straight parallel lines outward. We know that
y = 1/4p x2
so that
2 = 1/4p (1.5)2
Solving gives
8p = 2.25 or p = 0.28125 inches
Exercise: Frying an Insect
Suppose that you have a magnifying glass that is 3 inches in diameter and
.5 inched deep. How high above the ground should you hold the magnifying
glass so that it burns a hole in a leaf on the ground?
Back to the College Algebra Part II (Math 103B) Site
Back to the LTCC Math Department Page
e-mail Questions and Suggestions