Practice Midterm II Key

Problem 1

The South Lake Tahoe Police Department has charted the number of arrests over the past twelve months.  The number of arrests per month that are made follows a Normal distribution with mean 72 and standard deviation 12.  Construct a control chart and determine any out of control signals.

Solution

We first find 

        m + s  =  84        m + 2s  =  96        m + 3s  =  108

        m - s  =  60        m - 2s  =  48        m - 3s  =  36

Now we sketch the control chart

We can see that there are two out of control signals.  The first signal is that the seventh month was three standard deviations above the mean.  The second signal is that there are nine data points in a row on the same side of the mean line.

Problem 2

Stellar Jay hatchlings have a body weight that is approximately normally distributed with a mean of 3.4 ounces and a standard deviation of 0.3 ounces.  

A.  Convert the following x-intervals into z-intervals.

            x > 3.0                        x < 6.0

Solution

We find the z-score for each of these.  For the first one, we get

                       3.0 - 3.4
          z  =                            =  -1.33                
                           0.3

The z-interval is     z > -1.33

For the second one we find the z-score

                       6.0 - 3.4
          z  =                            =  8.67            
                           0.3

The z-interval is     z < 8.67

B.  Convert the following z-interval into an x-interval

            -1.2 < z < 0.6            

Solution

For this one, we go backwards to find the raw score.  First for z  =  -1.2, we have

                           x - 3.4
          -1.2  =                              
                             0.3

Multiply both sides by 0.3 to get

        -.36  =  x - 3.4

Add 3.4 to both sides to get

        x  =  3.04

Now we will work on z  =  0.6.

                            x - 3.4
          0.6   =                               
                              0.3

Multiply both sides by 0.3 to get

        0.18  =  x - 3.4

Add 3.4 to both sides to get

        x  =  3.58

Finally, we put this all together to get

        3.04 < x < 3.58

Problem 3

Your tire company's snow and mud tires have an average lifetime of 80,000 miles with a standard deviation of 10,000 miles.  Answer the following assuming the distribution is normal.

A.  If the current guarantee for the tires is 65,000 miles, about what percentage of the tires will wear out before the guarantee expires?

Solution

We first compute the z-score for this information.  We have

                     65,000 - 80,000
          z  =                                    =  -1.5                
                             10,000

We want the probability P(z < -1.5).  We go to the table to get a probability of 0.0668.  Compare our graph with the table graph and notice that this is the probability that we want.  We can say that about 6.7% of the tires will wear out before the guarantee expires.

B.  You want to reconsider the guarantee so that about 98% last past the guarantee period.  What should you set as the guarantee period on your tires?

Solution

This problem asks us to go backwards.  We want to find y such that P(x > y ) = 0.98.  The picture shows that we need to subtract from 1, that is we want to first find the z-score such that this z-score corresponds to a probability of .02.  The table gives us 

        z  =  -2.05

Next we work backwards to find the raw score.  We have

                               x - 80,000
          -2.05   =                                    
                                10,000

Multiply both sides by 10,000 to get

        -20,500  =  x - 80,000

Add 80,000 to both sides to get

        x  =  59,500

We should offer a 59,500 mile guarantee so that about 98% of the tires will last past the guarantee period.  

Problem 4

The Lake Tahoe Visitor's Authority has determined that 65% of the tourists who come to the Lake Tahoe area to go snowboarding are from the Bay area.  The Boarder Motel has all of its 35 rooms booked during this weekend.

A.  What is the probability that between 20 and 25 of the rooms host bay area visitors?

Solution

We first find the mean and standard deviation.  The formula gives 

        m  =  (35)(.65)  =  22.75

and

The continuity correction tells us that we want to find

        P(19.5 < x < 25.5)

Now we are ready to compute the z-scores.  We have

                            19.5 - 22.75
          z_19.5  =                                =  -1.15              
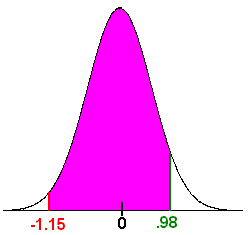
                                  2.82

and

                            25.5 - 22.75
          z_25.5  =                                =  0.98            
                                 2.82

The table gives us corresponding probabilities

        .1251        and        .8365

We want P(-1.15 < z < 0.98).  The picture indicates that we need to subtract these probabilities.  We get

        .8365 - .1251  =  .7114

We can conclude that there is about a 71% chance that between 20 and 25 of the visitors will be from the Bay Area.

B.  Why is your estimate valid?

Solution

We compute np and nq.  We have

        np  =  35(.65)  =  22.75        nq  =  35(.35)  =  12.25

since both of these value are greater than 5, we can conclude that the distribution is approximately normal and our calculations are valid.

Problem 5

 Explain what the difference is between a sampling distribution and the distribution of a sample.

Solution

A sampling distribution is the distribution of all possible samples of a fixed size taken from a population, while the distribution of a sample is the results that occur from only one individual sample that was taken.

Problem 6

It is know that the mean number of houses a Trick-Or-Treater visits is 46 and the standard deviation is 8.  

A.  Assuming that the distribution is approximately normal, what is the probability that your seven year old neighbor will visit fewer than 42 houses on Halloween?

Solution

We want P(x < 42).  We convert this to a z-score to get

                   42 - 46    
        z  =                      =    -0.5    
                         8

The corresponding probability that we get from the table is

        .3085

Since our graph matches the table graph, we can conclude that there is about a 31% chance that the child will visit fewer than 42 houses.

B.  25 children were randomly selected and observed.  What is the probability that their mean number of visits is between  48 and 55?

Solution

We compute the z-scores using the sampling distribution.  We have

The probability that corresponds with 1.25 is .8944.  Notice that 5.63 is off the chart.  Thus, the probability that corresponds with 5.63 is 1.  Since we want the "in between" area, we take 

        1 - .8944  =  .1056

We can conclude that there is about an 11% chance that the mean number of visits for these 25 children will be between 48 and 55.

Problem 7

Do you favor allowing pilots to carry a gun in the cockpit?  74% of Americans are in favor of allowing pilots to carry a gun in the cockpit. 

A.  80 passengers board a plane heading toward New York.  What is the probability that the greater than 75% of them favor allowing the pilot to carry a gun?

Solution

We want P(p > 0.75) however, we must first use the continuity correction.  We need 

        P(p > 0.75 + 0.5/80)  =  P(p > .756)

We find the z-score

The probability that corresponds with 0.33 is .6293.  Since we want the right hand side, we must subtract from 1.  We get

        1 - .6293  =  .3707

We can conclude that there is a 37% chance that the greater than 75% of the passengers will favor the pilot carrying a gun.

B.  Is the normal approximation to the proportion p = r/n valid?  Explain.

Solution

We need to determine if np and nq are greater than 5.  We have

        np  =  (80)(.74)  =  59.2            nq  =  (80)(.26)  =  20.8

Since these are both greater than 5, we can conclude that normal approximation is valid.

Problem 8

The manager of Wasabi restaurant tallied the number of customers that he received over a 50 day period.  He found that the mean number per day for this period was 45 with a standard deviation of 8.  Construct a 95% confidence interval for the true mean.  Write a sentence that explains your findings.

Solution

We find the margin of error first.  We have

        x  =  45        s  =  8        z_.95  =  1.96      n  =  50

We have

The confidence interval is 

        45  2.28

or 

        [42.72, 47.28]

We can conclude that there is a 95% chance that the true mean number of customers that visit Wasabi restaurant per day lies between 42.78 and 47.22.

Problem 9

Thirteen brown bears in the Sierra Nevada Mountains were captured and released for a research project.  Their mean weight was found to be 320 pounds with a standard deviation of 23 pounds.  

A.  Determine a 95% confidence interval for the mean weight of brown bears in the Sierra Nevada Mountains.

Solution

Notice that the sample size is small.  We use t_c instead of the z_c.  There are 

        13 - 1  =  12    degrees of freedom

The table gives 

        t_c  =  2.179

Now we can compute the margin of error

The confidence interval is 

        320 13.9

or

        [306.1, 333.9]

B.  What assumption do you need for your answer in part A to be valid?

Solution

We assumed that the bears were randomly selected and that the weight distribution is approximately normal.

C.  Write a sentence that explains your findings.

Solution

We are 95% confident that the mean weight for all Sierra Nevada black bears is between 306.1 and 333.9.

Problem 10

A psychologist is doing research on blindly following orders.  200 volunteers were ordered to push a button that would inflict 50 volts of electricity into a laboratory animal.  35 of them refused to push the button.  Construct a 90% confidence interval for the true proportion of people who will refuse to zap the animal.  Write a sentence that explains your findings.

Solution

We are asked to find a confidence interval for a proportion.  We have

        p   =  35/200  =  0.175        q  =  1 - .175  =  .825        n  =  200        z_c  =  1.645

We plug these numbers into the formula for the margin of error

The confidence interval is 

        .175  .044  

or

        [.131, .219]

We can conclude that there is a 90% chance that between 13% and 22% of all people will refuse to zap the animal when ordered.

Problem 11

Nationally, 2% of the population carry a venereal disease.  You are interested in constructing a 95% confidence interval for the mean number of carriers in the Tahoe Basin.  How many people will you need to test if you want a margin of error of  1%?

Solution

We need to find n.  Since we have information about the proportion, we can use p  =  .02.  We have E  =  .01.  We find

        n  =  p(1 - p)(z_c / E)²  =  (.02)(.98)(1.96 / .01)²  =  752.95

We round up so that we need to test 753 people.

Problem 12

A study was done to compare the pass rates of Caucasians and Latinos in their statistics class.  192 Caucasians and 83 Latinos were considered.  135 of the Caucasians and 70 of the Latinos passed the course.  Find a 95% confidence interval for p₁ - p₂ and explain in a complete sentence what it means.

Solution

We have

        p₁  =  135/192  =  .70        p₂  =  70/83  =  .84        n₁  =  192        n₂  =  83

We calculate

and conclude that a 95% confidence interval is 

        .14  .1

or

        [.04, .24]

(Notice that we put the larger one first)  We can conclude with a confidence level of 95% that the pass rates between Caucasians and Latinos differ by as little as 4% and as much as 24%.

Problem 13

Suppose that you were told that the mean number of hours that people watch television per day is 3.4.  You believe that this number must be lower and want to challenge this statement.  What would you use for the null hypothesis and the alternative hypothesis?  Sketch a picture that displays the critical region.

Solution

We have 

        H₀ :  m  =  3.4        H₁ :  m  <  3.4