Comparing, Ordering and Rounding Decimals
- Comparing Decimals
At the beginning of this course, we we encountered the number line, a graphical device that helps us visualize the relationships between two numbers. Just as we can place whole numbers on the number line, we can also place decimals on the number line. If two numbers are on a number line, then the number to the right is the larger one.
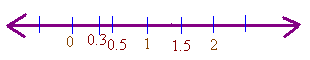
We see that that
0.5 < 2 and 0.3 < 0.5
To compare two decimals without placing them on a number line, follow the following method:
- Start from left to right and compare
corresponding digits. If the digits are the same, move to the
right.
- If the digits are different then the larger
number is the one with the larger digit.
Example
Fill in the blank with a "<", ">", or an "=" sign
- 3.1714
3.169
Solution
We see that the ones digits, 3 and 3, are the same, the tenths digit, 1 and 1 are the same, but the hundredths digits, 7 and 6, are different with 7 > 6. Hence the inequality is ">"
3.1714 > 3.169
- 0.0005
0.0023
Solution
We see that the ones digits, the tenths digits, and the hundredths digits are all 0. The left hand side has 0 for the thousandths digit while the right hand side has 2 for its thousandths digit. Hence the inequality is "<"
0.0005 < 0.0023
Exercises
Fill in the blank with a "<", ">", or an "=" sign
- 34.916
34.924

- 18.126
18.1260

- 123.437
123.337

Example
Place the following decimals from in order from smallest to largest
2.753 2.75 2.357 3 2.7
SolutionWe first add zeros to the ends of the shorter decimals to make comparison easier
2.753 2.750 2.357 3.000 2.700
Now we see that the smallest is 2.357, since its tenths digit, 5, is smaller than 5. Next is 2.700, since its hundredths digit, 0, is smaller than 5. Next comes 2.750 since its thousandths digit, 0, is smaller than 3.
Example
Place the following decimals from in order from smallest to largest
49.1 49.16 49.31 27 49.01

- Start from left to right and compare
corresponding digits. If the digits are the same, move to the
right.
Rounding Decimals to a Specified Decimal Place
It is often necessary to round a decimal to the nearest tenth, hundredth, thousandth, etc. When dealing with money we usually round to the nearest hundredth so that we can read the number in dollars and cents. The rules of rounding decimals are the same as the rules for rounding whole numbers. We look at the digit to the right and determine if it is 5 or greater. If it is greater than 4, round up. Otherwise round down.
ExampleRound 32.537 to the nearest hundredth.
Solution
The digit to the right of the hundredth place is "7". Since 7 is greater than 4, we round up. Change the 3 to a 4. We write
32.54
Example
Round 27.8149 to the nearest tenth.
Solution
The digit to the right of the tenth place is "1". Since 1 is not greater than 4, we round down. Keep the 8 the same. We write
27.8
Exercise
Round the following numbers as indicated.
651.955 to the nearest tenth

49.942 to the nearest hundredth

Back to the Math Department page
e-mail Questions and Suggestions