Key to Practice Exam 2
Please work out each of the given problems. Credit will be based on the steps that you show towards the final answer. Show your work.
Problem 1
The number of people y in town who will buy a Lake Tahoe sweatshirt if it is offered at x dollars can be modeled by the equation
50x + y = 2000
A. Find the y-intercept and interpret this number in the context of sweatshirt purchases.
Solution
To find the y-intercept, set x equal to zero and solve for y:
50(0) + y = 2000
y = 2000
We can interpret this by saying that if the sweatshirts are given out for free ($0) then 2000 residents will take it.
B. Find the x-intercept and interpret this number in the context of sweatshirt purchases.
Solution
To find the x-intercept, set y equal to zero and solve for x:
50x + 0 = 2000
50x = 2000
Now divide both sides by 50 to get
x = 40
We can interpret this by saying that when the price reaches $40 no (0) Tahoe residents will buy the sweatshirt.
C. Find the slope and interpret this number in the context of sweatshirt purchases.
Solution
We put the equation into y intercept form by subtracting 50x from both sides:
y = -50x + 2000
Now, we can read off the slope as
m = -50 = -50/1
We can interpret this by saying that for every $1 increase in the price, there will be 50 fewer sweatshirts sold. (as x increases by 1, y decreases by 50)
Problem 2
Sketch the graphs of the following lines and find the x and y intercepts is they exist.
A. 2x - 5y = 10
Solution
We find set x = 0 and find y:
2(0) - 5y = 10
-5y = 10 Divide both sides by -5
y = -2
Next set y = 0 and find x
2x - 5(0) = 10
2x = 10
x = 5
This gives the table
| x | y |
| 0 | -2 |
| 5 | 0 |
The plot the points and connect with a line. The x-intercept is 5 and the y-intercept is -2. The graph is shown below.
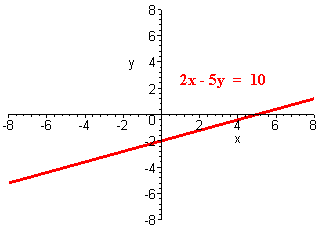
B. y = 4
Solution
This is just the horizontal line four units above the x-axis. There is no x-intercept and the y-intercept is 4.

C. y + 2/3 x = 4
Solution
We can subtract the 2/3 x from both sides to get
y = -2/3 x + 4
which is a line with slope -2/3 and y-intercept 4. We can begin at the point (0,4), "rise" down 2 and "run" across 3. To find the x-intercept, set y = 0 and solve.
0 = -2/3 x + 4
0 = -2x + 12
2x = 12
x = 6
The x-intercept is 6.
The graph is shown below.

D. x = -3
Solution
This is the vertical line that crosses the x-axis at x = -3. The x-intercept is -3 and there is no y-intercept. The graph is shown below.

E. The line that passes through the point (2,4) and has slope -3/2.
Solution
We just sketch the point (2,4) then "rise" down 3 and "run" across 2. The graph is shown below.
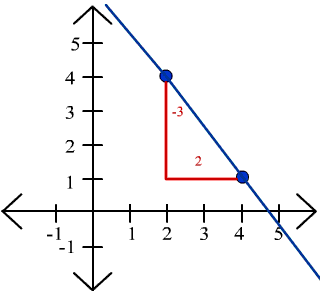
To find the intercepts, first find the equations using the point-slope formula.
(y - 4) = -3/2(x - 2)
y - 4 = -3/2 x + 3
y = -3/2 x + 7
We see that the y-intercept is 7. For the x-intercept, set y = 0 and solve.
0 = -3/2 x + 7
0 = -3x + 14
3x = 14
x = 14/3
So the x-intercept is at x = 14/3.
Problem 3
A. Find the slope of the line that is parallel to the line that passes through the points (2,-3) and (5,6).
Solution
Parallel lines have the same slope, so we need only to find the slope through the points (2,-3) and (5,6).
The rise is:
6 - (-3) = 6 + 3 = 9
and the run is:
5 - 2 = 3
Hence the slope is
m = rise/run = 9/3 = 3
B. Find the slope of the line that is perpendicular to the line that passes through the points (0,2) and (2,3).
Solution
First, we find the slope of the line through the two points.
The rise is:
3 - 2 = 1
and the run is:
2 - 0 = 2
Hence the slope is
m = rise/run = 1/2
Our line is perpendicular, so we take the reciprocal of the this slope and multiply by (-1) to get
(-1) (2/1) = -2
Problem 4
Find the equation of the line in slope intercept form with the following properties.
A. Passes through the points (2,3) and (4,-1).
Solution
First we find the slope. The rise is
-1 - 3 = -4
and the run is
4 - 2 = 2
Hence the slope is
m = rise/run = -4/2 = -2
Now use the point slope formula with the point (2,3) and the slope -2 to get
y - 3 = (-2)(x - 2) = -2x + 4 Add 3 to both sides
y = -2x + 7
B. Passes through the point (-4,2) and has slope -2/5.
Solution
We use the point slope formula to get
y - 2 = (-2/5)(x - (-4)) =((-2/5)(x + 4) = -2/5 x - 8/5 Add 2 to both sides
y = -2/5 x - 8/5 + 2 = -2/5 x - 8/5 + 10/5 = -2/5 x + 2/5
or
y = -2/5 x + 2/5
Problem 5
The graphs of several lines are shown below. decide which of the statements are true and which are false and explain your reasoning.

A. The slope of line II is greater than the slope of line I.
Solution
False. The slope of line II is negative and the slope of line I is positive. Negative numbers are always smaller than positive numbers.
B. The y-intercept of lines I and II are equal.
Solution
True. They are both equal to 2.
C. The x-coordinate of the x-intercept of line I is greater than the x-coordinate of the x-intercept of line III.
Solution
False. Line I has negative x-intercept and line III has negative x-intercept.
D. Lines I and II are perpendicular.
Solution
False. The slope of line I is 2/2 = 1 and the slope of line II is -2/1 = -2. Since their produce is
(1)(-2) = -2
which is not -1 they are not perpendicular.
Problem 6 Perform the indicated operations.
A. (3v4 - 5v3 + 9v - 1) + (2v4 + 7v2 - 8v - 3)
Solution
We just combine like terms. Notice that we can drop the parentheses, since we are adding:
3v4 - 5v3 + 9v - 1 + 2v4 + 7v2 - 8v - 3
= 3v4 + 2v4 - 5v3 + 7v2 + 9v - 8v - 1 - 3
= 5v4 - 5v3 + 7v2 + v - 4
B. (2x5 - x4 - 5x3 + 3x2 - 6) - (4x5 - x4 - 2x3 + 3x2 - 7x - 10)
Solution
We first distribute the "-" through by changing all of the signs of the second expression
= 2x5 - x4 - 5x3 + 3x2 - 6 - 4x5 + x4 + 2x3 - 3x2 + 7x + 10
Now combine like terms
= 2x5 - 4x5 - x4 + x4 - 5x3 + 2x3 + 3x2 - 3x2 + 7x - 6 + 10
= -2x5 - 3x3 + 7x + 4
Problem 7 Find each product
A. 3x8yz2(5xy2 + 3x3z2 - 6y + 1)
Solution
We use the distributive property (multiply through) and use the product rule for exponents:
= 15x8+1 y1+2 z2 + 9x8+3 y z2+2 - 18x8y1+1z2 + 3x8yz2
= 15x9y3z2 + 9x11yz4 - 18x8y2z2 + 3x8yz2
B. (2x - 3)(x3 + 4x - 10)
Solution
Use the distributive law. First with the 2x and then with the -3:
= 2x(x3 + 4x - 10) + (-3)(x3 + 4x - 10)
= 2x4 + 8x2 - 20x - 3x3 - 12x + 30
Now combine like terms
= 2x4 - 3x3 + 8x2 - 20x - 12x + 30
= 2x4 - 3x3 + 8x2 - 32x + 30
Problem 8 Find each product
A. (2x + 9)(3x - 1)
Solution
Use FOIL
= (2x)(3x) + (2x)(-1) + (9)(3x) + (9)(-1)
= 6x2 - 2x + 27x - 9
Now combine like terms
= 6x2 + 25x - 9
B. (3x - 1/2)(4x - 2/3)
Solution
Use FOIL
= (3x)(4x) + (3x)(-2/3) + (-1/2)(4x) + (-1/2)(-2/3)
= 12x2 - 2x - 2x + 1/3
Now combine like terms
= 12x2 - 4x + 1/3
C. (x + 1)(x + 2)(x + 3)
Solution
Use FOIL on the first two factors
(x + 1)(x + 2) = x2 + 2x + x + 2 = x2 + 3x + 2
Now multiply this by the third term
(x + 3)(x2 + 3x + 2) = (x)(x2 + 3x + 2) + 3(x2 + 3x + 2)
= x3 + 3x2 + 2x + 3x2 + 9x + 6
Now combine like terms
= x3 + 6x2 + 11x + 6
Problem 9 Use the special product formulas to find
A. (3x - 4)2
Solution
This is the square of a binomial. We have
(3x)2 + 2(3x)(-4) + (-4)2
= 9x2 = 24x + 16
B. (7z - 3)(7z + 3)
Solution
This is the difference of squares. We have
= (7z)2 - (3)2
= 49z2 - 9
Problem 10 Simplify
A. (x2y5x4)10
Solution
First use the product rule on the x's.
= (x2+4y5)10 = (x6y5)10
Use the power rule.
= x(6)(10)y(5)(10)
= x60y50
B. -20(a2b)7
(2ab5)2a5
Solution
First use the power rule (multiply the 7 through the top and the 2 through the bottom.
-20a14b7
=
4a2b10a5
Now use the product rule on the a's.
-20a14b7
=
4a7b10
Now use the quotient rule.
-5a7
=
b3
Problem 11 Simplify and write without negative exponents.
(x-3 y) -2(x-4)
Solution
First use the power rule
= (x6 y-2)(x-4)
Now use the product rule
= x2 y-2
Now remember that we can write without negative exponents.
x2
=
y2
Problem 12 The Milky Way galaxy is about 120,000 light years across. One light year is 950,000,000,000,000 meters long.
A. Write both of these numbers in scientific notation.
Solution
The first number is
1.2 x 105
and the second number is
9.5 x 1014
B. How many meters wide is the milky way galaxy? Write your answer in scientific notation.
Solution
We just multiply the two numbers.
(1.2 x 105)(9.5 x 1014) = (1.2)(9.5)(105)(1014)
= 11.4 x 1019 = 1.14 x 101 x 1019 = 1.14 x 1020
e-mail
Questions and Suggestions