Practice Exam 2

Practice Exam 2

Please work out each of the given problems. Credit will be based on the steps that you show towards the final answer. Show your work.

Problem 1

The number of people y in town who will buy a Lake Tahoe sweatshirt if it is offered at x dollars can be modeled by the equation

50x + y = 2000

A. Find the y-intercept and interpret this number in the context of sweatshirt purchases.

Solution

To find the y-intercept, set x equal to zero and solve for y:

50(0) + y = 2000

y = 2000

We can interpret this by saying that if the sweatshirts are given out for free ($0) then 2000 residents will take it.

B. Find the x-intercept and interpret this number in the context of sweatshirt purchases.

Solution

To find the x-intercept, set y equal to zero and solve for x:

50x + 0 = 2000

50x = 2000

Now divide both sides by 50 to get

x = 40

We can interpret this by saying that when the price reaches $40 no (0) Tahoe residents will buy the sweatshirt.

C. Find the slope and interpret this number in the context of sweatshirt purchases.

Solution

We put the equation into y intercept form by subtracting 50x from both sides:

y = -50x + 2000

Now, we can read off the slope as

m = -50 = -50/1

We can interpret this by saying that for every $1 increase in the price, there will be 50 fewer sweatshirts sold. (as x increases by 1, y decreases by 50)

Problem 2

The length of a rectangle is 18 inches less than three times its width. The perimeter of the rectangle is 52 inches. Find the dimensions of the rectangle.

Solution

We draw the picture below

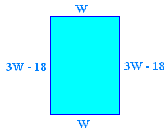
We let

W = the width of the rectangle

then

3W - 18 = the length of the rectangle

The perimeter is the sum of twice the length and twice the width.

2(3W - 18) + 2W = 52

6W - 36 + 2W = 52

8W - 36 = 52

Now add 36 to each side.

8W = 88

Next divide by 4 to get

W = 11

so that the length is

3(11) - 18 = 33 - 18 = 15

The rectangle has width 11 and length 15.

Problem 3

To buy your $25,000 car, you had to get loans from two different sources for the $25,000. The first source charged 6% interest and the second source charged 8% interested per year. If the total amount of interest that you will pay for the first year is $1800, how much did you borrow from each source?

Solution

Let x = the loan amount at 6% interest

25000 - x = the loan amount at 8% interest

Then

0.06x + 0.08(25000 - x) = 1800

Multiply by 100 to clear the decimals:

6x + 8(25000 - x) = 180000

Now distribute the 8

6x + 200000 - 8x = 180000

Combine like terms to get

-2x + 200000 = 180000

Subtract 200000 from both sides to get

-2x = -20000

Now divide by -2

x = 10000

We can conclude that the loan amount at 6% was $10,000 and the loan amount at 8% interest was $15,000.

Problem 4

Sketch the graphs of the following lines and find the x and y intercepts is they exist.

A. 2x - 5y = 10

Solution

We find set x = 0 and find y:

2(0) - 5y = 10

-5y = 10 Divide both sides by -5

y = -2

Next set y = 0 and find x

2x - 5(0) = 10

2x = 10

x = 5

This gives the table

x	y
0	-2
5	0

The plot the points and connect with a line. The x-intercept is 5 and the y-intercept is -2. The graph is shown below.

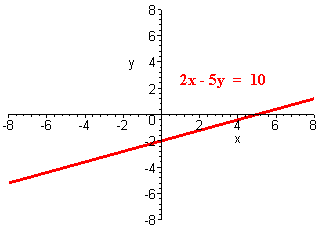
B. y = 4

Solution

This is just the horizontal line four units above the x-axis. There is no x-intercept and the y-intercept is 4.

C. y + 2/3 x = 4

Solution

We can subtract the 2/3 x from both sides to get

y = -2/3 x + 4

which is a line with slope -2/3 and y-intercept 4. We can begin at the point (0,4), "rise" down 2 and "run" across 3. To find the x-intercept, set y = 0 and solve.

0 = -2/3 x + 4

0 = -2x + 12

2x = 12

x = 6

The x-intercept is 6.

The graph is shown below.

D. x = -3

Solution

This is the vertical line that crosses the x-axis at x = -3. The x-intercept is -3 and there is no y-intercept. The graph is shown below.

E. The line that passes through the point (2,4) and has slope -3/2.

Solution

We just sketch the point (2,4) then "rise" down 3 and "run" across 2. The graph is shown below.

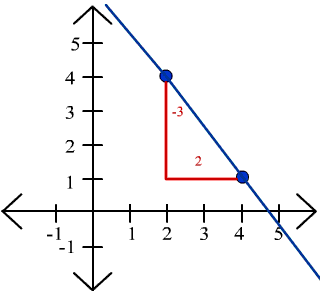
To find the intercepts, first find the equations using the point-slope formula.

(y - 4) = -3/2(x - 2)

y - 4 = -3/2 x + 3

y = -3/2 x + 7

We see that the y-intercept is 7. For the x-intercept, set y = 0 and solve.

0 = -3/2 x + 7

0 = -3x + 14

3x = 14

x = 14/3

So the x-intercept is at x = 14/3.

Problem 5

A. Find the slope of the line that is parallel to the line that passes through the points (2,-3) and (5,6).

Solution

Parallel lines have the same slope, so we need only to find the slope through the points (2,-3) and (5,6).

The rise is:

6 - (-3) = 6 + 3 = 9

and the run is:

5 - 2 = 3

Hence the slope is

m = rise/run = 9/3 = 3

B. Find the slope of the line that is perpendicular to the line that passes through the points (0,2) and (2,3).

Solution

First, we find the slope of the line through the two points.

The rise is:

3 - 2 = 1

and the run is:

2 - 0 = 2

Hence the slope is

m = rise/run = 1/2

Our line is perpendicular, so we take the reciprocal of the this slope and multiply by (-1) to get

(-1) (2/1) = -2

Problem 6

Find the equation of the line in slope intercept form with the following properties.

A. Passes through the points (2,3) and (4,-1).

Solution

First we find the slope. The rise is

-1 - 3 = -4

and the run is

4 - 2 = 2

Hence the slope is

m = rise/run = -4/2 = -2

Now use the point slope formula with the point (2,3) and the slope -2 to get

y - 3 = (-2)(x - 2) = -2x + 4 Add 3 to both sides

y = -2x + 7

B. Passes through the point (-4,2) and has slope -2/5.

Solution

We use the point slope formula to get

y - 2 = (-2/5)(x - (-4)) =((-2/5)(x + 4) = -2/5 x - 8/5 Add 2 to both sides

y = -2/5 x - 8/5 + 2 = -2/5 x - 8/5 + 10/5 = -2/5 x + 2/5

y = -2/5 x + 2/5

Problem 7

The graphs of several lines are shown below. decide which of the statements are true and which are false and explain your reasoning.

A. The slope of line II is greater than the slope of line I.

Solution

False. The slope of line II is negative and the slope of line I is positive. Negative numbers are always smaller than positive numbers.

B. The y-intercept of lines I and II are equal.

Solution

True. They are both equal to 2.

C. The x-coordinate of the x-intercept of line I is greater than the x-coordinate of the x intercept of line III.

Solution

False. Line I has negative x-intercept and line III has negative x-intercept.

D. Lines I and II are perpendicular.

Solution

False. The slope of line I is 2/2 = 1 and the slope of line II is -2/1 = -2. Since their produce is

(1)(-2) = -2

which is not -1 they are not perpendicular.

Problem 8

Solve the following inequalities and sketch the solution set on a number line.

A. 3x - 5 > 2

Solution

3x - 5 > 2 add 5 to both sides

3x > 7 divide both sides by 3

x > 7/3

The graph is shown below.

B. 5 - 2x > 9

Solution

5 - 2x > 1 subtract 5 from both sides.

-2x > -4 divide by -2, changing the inequality sign for the negative.

x < 2

The graph is shown below.

C. -4 < 6 - 2x < 10

Solution

-10 < 6 - 2x < 10

-16 < -2x < 4 Subtract 6 from all three sides.

8 > x > -2 Divide all three by -2, changing the inequality sign for the negative.

The graph is shown below.

Problem 9

Susan earned 75% and 81% on the first two exams. If she wants the average, x, of the first three exams to be at least 80%, what are the possible scores that she can get on her exam? Write your answer in interval notation.

Solution

We set the average of the three greater than or equal to 80:

        75 + 81 + x
                                >        80
                3

Now both sides by 3 and notice that since 3 is a positive number, you do not need to change the inequality sign

75 + 81 + x > 240

156 + x > 240

Subtract 156 from both sides to get

x > 84

So Susan must earn at least a score of 84% in order to obtain an average of at least 80%.

e-mail Questions and Suggestions