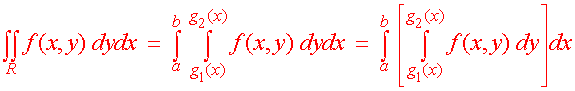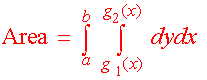Iterated Integrals and Area

 

Definition of an Iterated Integral

Just as we can take partial derivative by considering only one of the variables a true variable and holding the rest of the variables constant, we can take a "partial integral".  We indicate which is the true variable by writing "dx", "dy", etc.  Also as with partial derivatives, we can take two "partial integrals" taking one variable at a time.  In practice, we will either take x first then y or y first then x.  We call this an iterated integral or a double integral.

 

Definition of a Double Integral

Let f(x,y) be a function of two variables defined on a region R bounded below and above by 

                y  =  g1(x)          and          y  =  g2(x)

and to the left and right by

                x  =  a          and          x  =  b

then the double integral (or iterated integral) of f(x,y) over R is defined by

 

Example

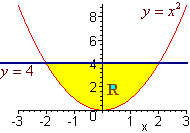
Find the double integral of  f(x,y)  =   6x2 + 2y  over R where R is the region between y  =  x2 and y  =  4. 

 

Solution

First we have that the inside limits of integration are x2 and 4.  The region is bounded from the left by x  =  -2 and from the right by x  =  2 as indicated by the picture below.

        

We now integrate

       

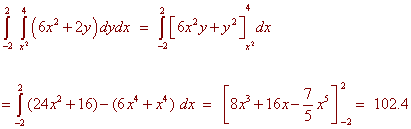
Changing the Order of Integration

If a region is bounded from the left by  x  =  h1(y) and the right by  x  =  h2(y) and below and above by y  =  c and y  =  d, then we can find the double integral of "dxdy" by first integrating with respect to x then with respect to y.  Sometimes there is a choice to make as to whether to integrate first with respect to x and then with respect to y.  We do whatever is easier.  

 

Example

Find the double integral of  f(x,y)  =  3y over the triangle with vertices (-1,1), (0,0), and (1,1).

       

Solution

If we try to integrate with respect y first, we will have to cut the region into two pieces and perform two iterated integrals.  Instead we integrate with respect to x first.  The region is bounded on the left and the right by x  =  -y  and x  =  y.  The lowest the region gets is y  =  0  and the highest is  y  =  1.  The integral is 

       

 

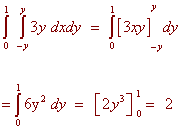
Example

Evaluate the integral

       

 

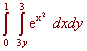
Solution

Try as you may, you will not find an antiderivative of and we don't want to get into power series expansions.  We have another choice.  The picture below shows the region.

       

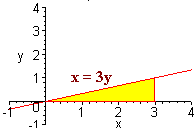
We can switch the order of integration.  The region is bounded above and below by y  =  1/3 x  and  y  =  0.  The double integral with respect to y first and then with respect to x is 

       

The integrand is just a constant with respect to y so we get

       

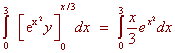
This integral can be performed with simple u-substitution. 

        u  =  x2        du  =  2x dx

and the integral becomes

       

Area

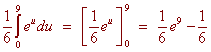
Recall from first year calculus, if a region R is bounded below by  y  =  g1(x)  and above by  y  =  g2(x), and  a  <  x  <  b, the area is given by 

       

There is another way of achieving this expression.  If we let the integrand by 1 then the double integral over the region R is 

       

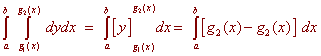
This gives us another way of finding area.  

Theorem:  Area and Double Integrals

 If a region R is bounded below by  y  =  g1(x)  and above by  y  =  g2(x), and  a  <  x  <  b, then the area is given by

              

Remark:  If the region if bounded on the left by x  =  h1(y) and the right by h2(y) with c <  y  <  d, then the double integral of 1 dxdy can also be used to find the area. 

 

Example 

Set up the double integral that gives the area between  y  =  x2  and  y  =  x3.   Then use a computer or calculator to evaluate this integral.

 

Solution

The picture below shows the region

       

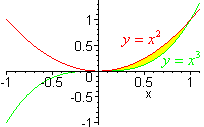
We set up the integral 

       

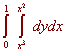
A computer gives the answer of 1/12.

 

 

Back to the Math 117 Home Page

Back to the Math Department Home

e-mail Questions and Suggestions