Work
Work
We define the work, W, done in moving an object a distance s units
with a force of F as:
| W = Fs |
Example: For me to jump 2 feet in the air, if I weigh 165 pounds, takes
W = Fs = 165(2) = 330 foot pounds.
Hook's Law
For a spring
| F = kd |
where d is the distance the spring is from its equilibrium point,
F is the
force exerted to compress to that distance, and k is a proportionality
constant.
Example
To compress a spring 2 inches from its equilibrium
point requires 50 pounds of force. How much work is it to compress
the spring an additional 2 inches?
Solution
We first find k:
50 = k(2)
hence
k = 25
We have
F = 25d
To find the work done, we cut the work into small
intervals of work. The force required to move the spring Dx units
is 25x, hence the work required is
Dw
= 25x Dx
Adding the small intervals, we have that
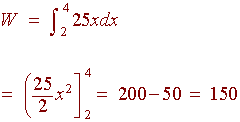
Example:
Sending a Rocket to Mars
Suppose that a 50 ton rocket is to be sent to mars and after
100,000 miles,
the rocket is completely free of the earth's gravity. If the radius
of the earth is 4,000 miles, how much work is required to send the rocket
out of orbit?
Solution
We use that the force of gravity is inversely proportional
to the square of the distance from the center of the earth. 
| F = k/x2 |
We have that
k
50 tons =
40002
or that
k = 800,000,000.
We have that
W = Fs
Cutting the work into small intervals and adding,
we have
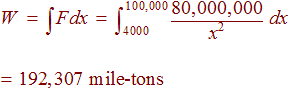
Emptying a Tank
Example
Suppose that a cylindrical tank of water with radius 10 and height
20 is
pumped out fully. How much work is required?
Solution:
We use the
formula
F =
weight = (density)(volume)
Considering cross-sections of the tank, we have
DF
= (64 lbs per cu ft)p
r2 Dh cu ft = 64p(100)Dy
The distance that water y feet above the ground must be
pumped is
(20 - y) feet.
We have
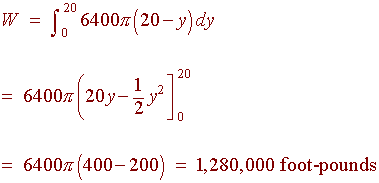
Lifting a Chain
Example
A 2,000 lb car has gone over a 100 foot cliff at the pass. A crane lifts up to car with a chain that is 10 lbs per foot. How much work is done?
Solution:
Work = Work for the car + Work for
the chain
We calculate:
Work for the car = 2,000(100)
and since the force is 10 times the distance the chain link is
from the top
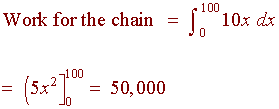
.
Hence the total work is
200,000 + 50,000 = 250,000 ft lbs.
Running an Automobile
An automobile is run by a piston which turns the wheel. We have the equation
Force = pressure (lbs/sq inch) times Volume ( cu inches) = (pressure)(p r2)
Boyle's Law states that the pressure is inversely proportional to the volume:
P = k/V
Hence
k
DW
=
p r2 Dx
V
But
p r2D
x = D V
Hence the total work is
![]()
Example
Suppose a piston has gas filled
initially to a volume of 0.5 cu ft and a pressure of
800 lbs/sq ft. The
piston expands to a volume of 1 cu ft. Find the work done by the gas.
Solution
We have
P = k/v
Plugging in gives
800 = k/0.5
So that
k = 400
Now we compute the work:
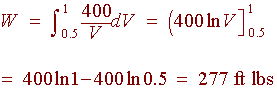
Back to the Math Department Home
e-mail Questions and Suggestions