Infinite Limits
Consider the function
f(x) = x/(x - 1)
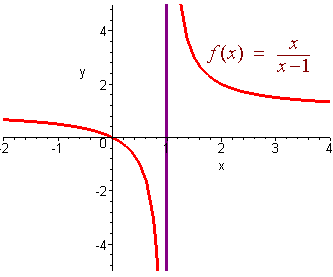
From the graph we see that there is a vertical asymptote at x = 1. We say
![]()
and
![]()
Formal Definition
Definition We say
if for
any choice of N there exists a
d such that whenever |
Example
Prove that
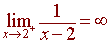
Solution
Let N be given then choose d such that
1/d > N
We have that if
x - 2 < d
then
1 1
>> N
x - 2 d
Hence the limit is infinity.
Other Sites on Limits
Weisstein's World of Mathematics