Asymptotes
Definition of a Limit at Infinity
Definition of a Limit at Infinity Let L be a real number and f(x) be a function. Then
if for every e > 0, there is an M > 0 such that |f(x) - L| < e whenever x > M. |
In other words as x gets
very large f(x) gets very close to
L.
If
![]()
then
we say that y = L is a horizontal asymptote of
f(x).
Example
Find
the horizontal asymptote of
x2 - 1
f(x) =
2x2 + x - 3
Solution:
Divide
by x2 on the numerator and the denominator to get
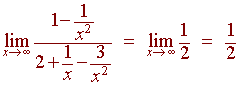
Horizontal Asymptotes of Non-Rational Functions
Example
Find the horizontal asymptotes of
![]()
Solution
We must consider the negative infinity case separately from the positive
infinity case. First note that for negative x,
![]()
hence
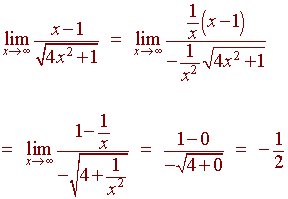
Next for positive,
![]()
hence
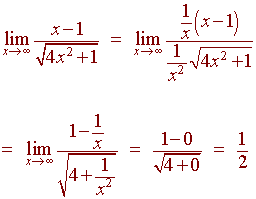
We see that there is a left horizontal asymptote at y = -1/2 and a right horizontal asymptote at y = 1/2.
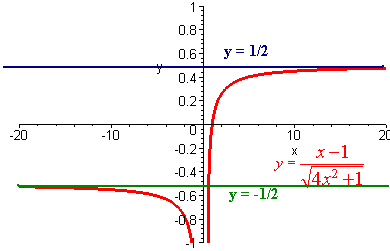
Example
Find the horizontal asymptotes of
sin x
f (x) =
x
Solution
We see that
1 sin
x 1
-
<
<
x
x
x
for all x. Both of the outer limits approach
0 as x approaches either
infinity or negative infinity. By the squeeze theorem, the middle
limit must approach zero. We can conclude that f has a horizontal
asymptote at y = 0.
e-mail Questions and Suggestions