ANTIDERIVATIVES

Definition

Suppose that F'(x) = f(x).  Then F(x) is called an antiderivative of f(x). 

 Examples

1. An antidervative of sin(x) is -cos(x).
   

2. An antiderivative of x² is 1/3 x³
   

Differential Equations  

Suppose we want to find the solution to

        dy/dx = f(x)

This is another way of asking for an antiderivative of f.  


Example

Find the solution to the differential equation 

        dy/dx = 3x² - 4x + 2


Solution

We find the antiderivative of 

        3x² - 4x + 2

We can find this antiderivative by finding the antiderivative of x², x, and 2 individually and then subtracting and adding.  We have

        

Function	x2	x	2
Antiderivative	1           x3    3	1          x2    2	2x

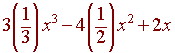
        =  x³ - 2x² + 2x

Notice that since the derivative of a constant is zero, adding a constant of an antiderivative results in another antiderivative for the same function.  We can write the final answer as

        x³ - 2x² + 2x + C

where C represents any constant.

Notation

The antiderivative of f(x) will be denoted by

Back to Math 105 Home Page

e-mail Questions and Suggestions